UFC 4-022-01
25 May 2005
The velocity at the second detector loop can be found to be 19.84 meter/sec = 71.4 km/hr (44.4
mph). The detection loop will alarm since the velocity exceeds the minimum setting. The
response time is equal to the sum of the barrier and security personnel reaction times, which
equals 7 seconds. Since the roadway is flat and straight, the distance traveled in 7 seconds can
1
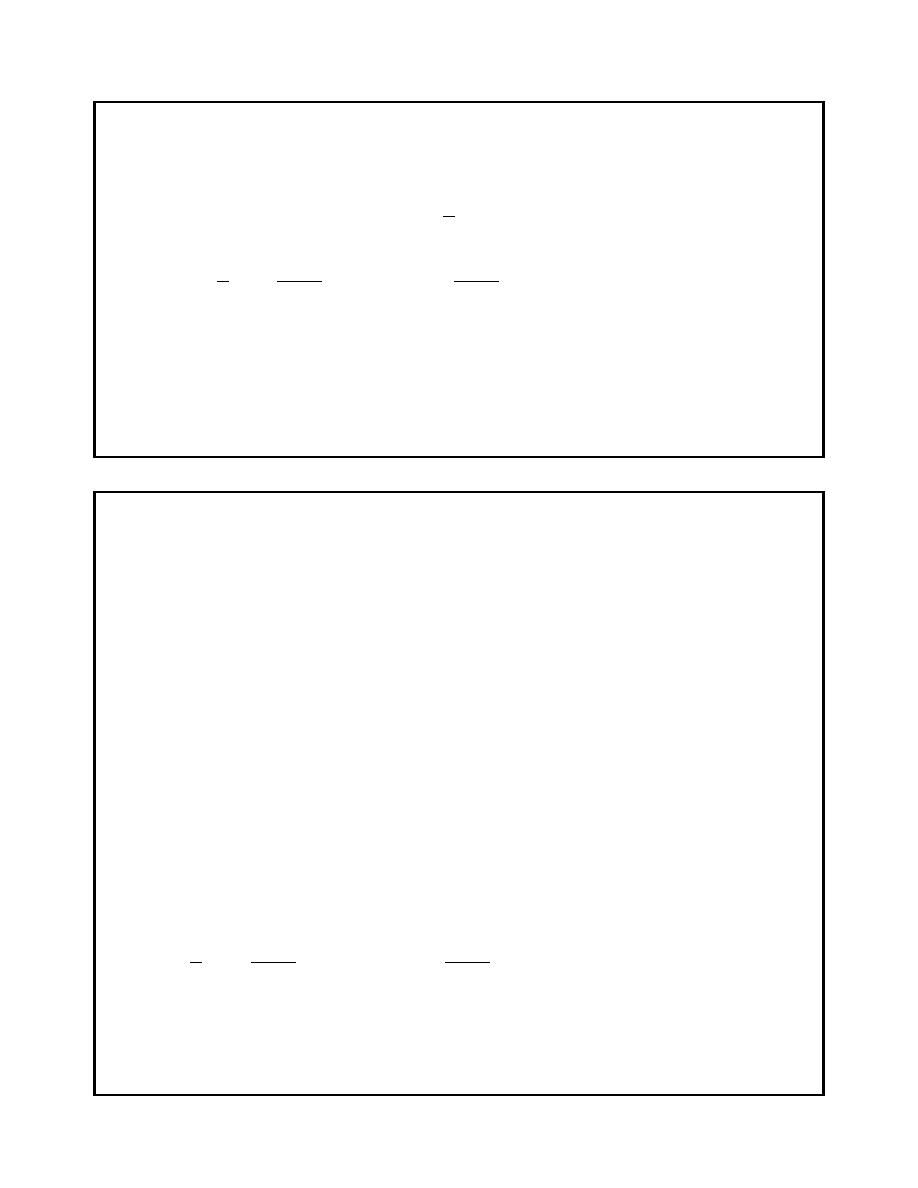
s=
⋅ ao ⋅ t 2 + vo ⋅ t where, s = distance traveled; t =
be calculated with the following formula:
2
1
meter
meter
Therefore, s =
⋅ (3.44
) ⋅ (7 sec)2 + (19.84
) ⋅ (7 sec) = 223.2meter = 732.3 feet
2
2
sec
sec
Since the attack is detected 30.5 meters prior to the checkpoint, the final minimum length of the
response zone is 223.2 meters 30.5 meters = 192.7 meters (632.3 feet).
If and there is an additional 4 second delay following the EFO being triggered for safety and
signals prior to the barrier deploying, the total response time is increased to 11 seconds. This
would increase the minimum length of the response zone to 396 meters (1300 feet).
Example 6-2:
Given:
Assume the conditions given in Example 6-1, with the addition of a S-curve or speed
control device in the response zone. The speed control area begins50 ft (15.24 m) past
the end of the access control zone and it limits the maximum velocity of vehicles to 25
mph (40 km/h).
The speed control device controls the speed of the vehicle for the first 150 ft (45.72 m) of
the response zone. The maneuvers added by the speed control device add 50 feet
(15.24 m) of additional path for the vehicle to traverse.
The threat vehicle is traveling at the maximum velocity allowed by speed control
measures
Solution:
The vehicle cannot enter the response zone at a speed greater than 40 km/h, therefore for the
first 200 ft (61 m), the vehicle will be traveling approximately 40 km/h. When the added length
of travel is added for maneuvering, the total distance traveled to reach the end of the speed
control area is 250 ft (76.2 m). At 40 km/h (11.2 m/s), it will take 6.8 seconds to reach the end
of the speed control area. Using the same formula as utilized in Example 6-1 the distance
traveled in the remaining 0.19 seconds until the response time is complete:
1
meter
meter
s=
⋅ (3.44
) ⋅ (0.19 sec) 2 + (11.2
) ⋅ (0.19 sec) = 2.19meters = 7.19 feet
2
2
sec
sec
Therefore the total distance traveled in the 7-second response time and the required length of
the response zone is 257.2 ft (78.39m). This is a 375 ft (114 m) reduction in the size of the
response zone compared to Example 6-1, achieved by using speed management immediately
following the access control zone.
6-8


 Previous Page
Previous Page
